2020. 3. 3. 02:33ㆍ카테고리 없음
Angular Momentum of a Rigid BodyAngular momentum of a rigid body ( Figure 1)can be obtained from the sum of the angular momentums of the particles formingthe body:1Figure1where r i = the position vectorof particle i, and w = the angular velocity vector of the rigid body. Now, let23Note here that a local reference frame, the XYZ system, isdefined and fixed to the body at O and r i& w aredescribed in this frame.
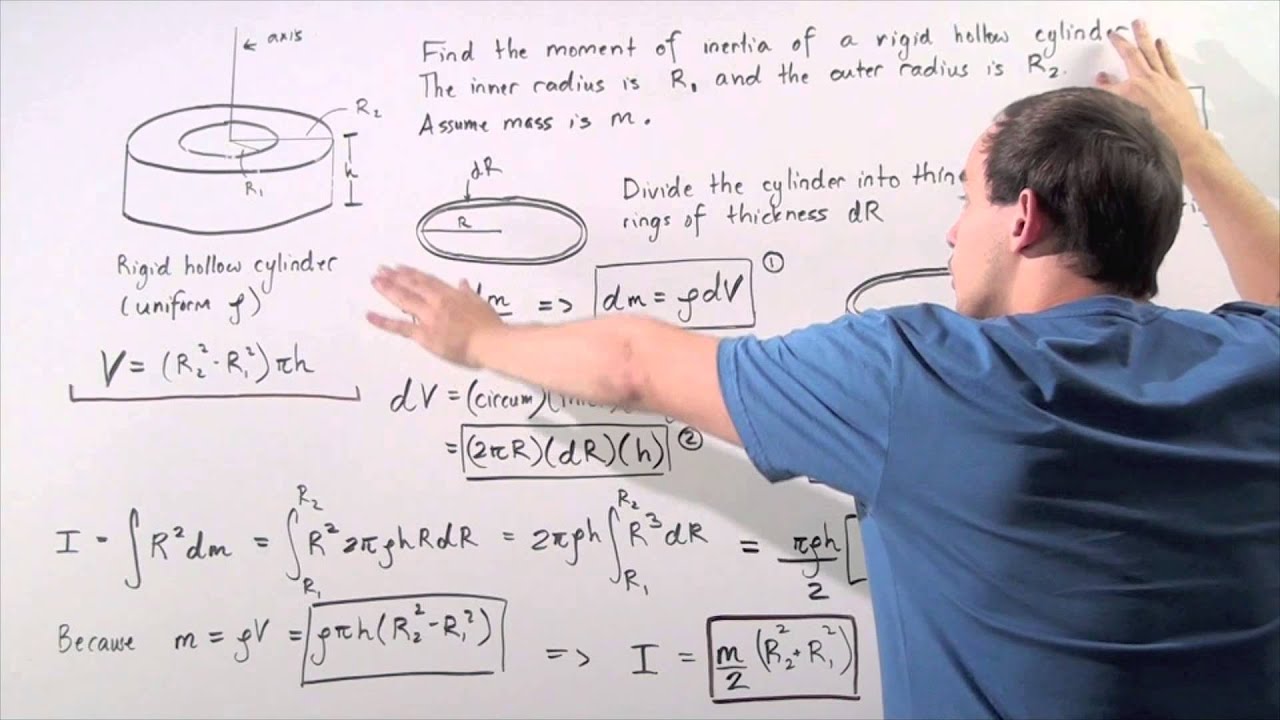
Moment Of Inertia Tensor For Cylinder

Substituting 2 & 3 into 1:4Inertia TensorLet5Substituting 5 into 4:67where I = the inertia tensor. The angular momentumof a rigid body rotating about an axis passing through the origin of the local referenceframe is in fact the product of the inertia tensor of the object and the angular velocity.The diagonal elements in the inertia tensor shown in 7, I xx,I yy & I zz, are called the moments ofinertia while the rest of the elements are called the products of inertia.Also see for moredetails of the moments and products of inertia. As shown in 7,the inertia tensor is symmetric.The 3 x 3 matrix in 7 suffices therequirements of a tensor of the 2nd rank:8where i, j, k & l = 1 to 3, t ij = an elementof the orthogonal transformation matrix, and I' ij = an element of thetransformed inertia tensor. This property is explained in detail in. In fact, a scalar is a tensor of zero rank:9where S = a scalar, S' = the transformed scalar.In addition, a vector is a tensor of the first rank since its transformation follows1010 is identical to 2 shown in. A 2-dimensional symmetric matrix is not necessarily a tensor of the 2nd rank.It must suffice 8 to be a tensor.© Young-Hoo Kwon, 1998.